Nanex Research

Nanex ~ 24-Jul-2012 ~ Sub-penny Price Anomaly

Update: 26-Jul-2012
We have completed a study of all sub-penny trades between 2006 and July 25, 2012. Make sure you read about the 3 different parties impacted by every sub-penny transaction below before clicking on this link.

Update: 25-Jul-2012

Here are some interesting facts we found after summing up data for every trading day over the last year (23-Jul-2011 to 24-Jul-2012):



We recently grouped all trade execution reports priced with 3 or more decimal places into 100 different bins by using the 2 digits that represent hundredths of a penny. We only include trades priced above $5 from NYSE, ARCA, and Nasdaq listed equities. For example, a trade with a price of 27.8099 is added to bin 99  (last 2 digits), while a trade with a price of 56.2520 is added to bin 20. We did this to see if anything interesting came out of the data (and found quite a surprise, see below).

Two columns of sub-penny price examples showing the hundredths place (becomes the bin number)
  

It helps to understand a few dynamics of how and why sub-penny trade executions occur. Dennis Dick over at PremarketInfo.com has written several excellent articles explaining sub-penny trade executions: Retail Price Improvement Scam, Exploring the Hidden Costs of Retail Price Improvement , and Dark Secrets: Where Does Your Retail Order Go? 

Expressing a price in pennies requires 2 decimals places (0.01) and prices that lie between 2 pennies (sub-penny) requires 3 or more decimal places. For example, the price 31.4601 lies between 31.46 and 31.47. Retail investors can only place orders priced to the nearest penny. But eligible market makers can enter orders priced to 4 decimal places, and often do this to give a retail order a "better" price.

It is important to understand that every trade that undergoes this price improvement process involves 3 parties and results in a trade execution price with more that 2 decimal places printed to the tape.  The 3 parties are:
  1. The retail investor (Investor A) whose order receives a slightly better price.
  2. The market maker who provided the slightly better price (we'll use the abbreviation HFT to refer to this party).
  3. The other retail investor (Investor B) whose order was not executed because HFT stepped ahead with the slightly better price.

The benefit to Investor A is obvious. The loss to Investor B is sometimes chalked up as a lost opportunity cost (missed trade execution), but is actually easy to calculate. We know the cost that Investor B would incur to ensure their trade executed at the time HFT stepped in front of their order - it's simply the best bid (if Investor B was selling) or the best offer (if they were buying). The best case scenario therefore would be the loss of the bid/ask spread which would be at least 1 cent per share. Likewise, we can simplify and say HFT benefits by the amount of Investor B's loss minus the price improvement to Investor A's trade (HFT will also capture exchange rebates, but for simplicity, we'll leave that out).  Basically, HFT and Investor A split a profit of a penny per share, the same one that was lost by Investor B (calculated from opportunity cost).

That leaves the question of how Investor A and HFT split the 1 cent profit. If we assume HFT will only part with the absolute minimum amount necessary, then trades executing just 1/100th of a cent away from the next penny will be split 99/100ths to HFT and 1/100th to Investor A. Likewise, prices 2/100ths of a cent away will be split 98/100ths to HFT and 2/100ths to the investor. This split continues all the way down to a price that is exactly halfway between two whole cents, such as 75.0050. Now these are special cases, because several brokers will execute retail orders meeting certain criteria at the mid-point of the bid-ask spread (which in many cases are priced in 1/2 cents). Therefore, we will exclude any trades priced exactly halfway between two whole cents, because we have no way of differentiating this group.

By using the criteria above, we can calculate the amount gained and lost by Investor A, HFT and Investor B, by simply grouping sub-penny trade executions using the last 2 digits (tenths and hundredths of a penny). This results in 99 bins (1 through 99). Bin 1 represents prices 0.0001 away from the nearest cent, and bin 99 also represents prices 0.0001 away from the nearest cent (12.2699 is 0.0001 away from 12.2700). Therefore we can combine bins 1 and 99, bins 2 and 98, bins 3 and 97 and so forth up to bins 49 and 51. That gives us 49 combined bins and one remaining bin 50. Since bin 50 represents prices exactly between cents, and these can result from other mechanisms, we'll exclude bin 50, leaving us with 49 bins.

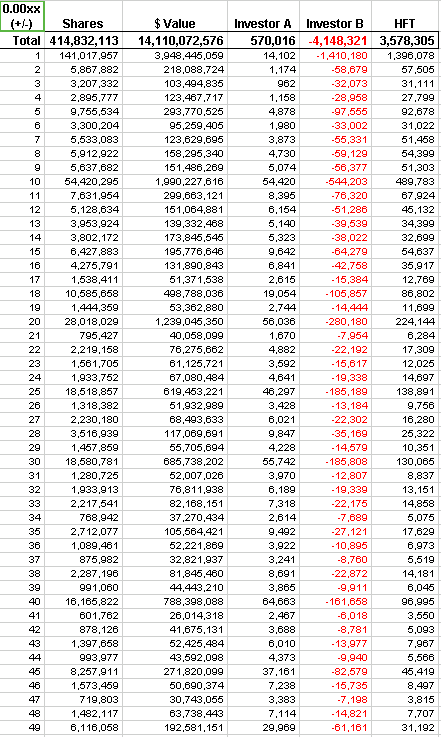
The image below shows the trades for July 19, 2012 grouped by these 49 bins. You can download the spread sheet here.

The 1st column indicates the bin. Bin 1 represents trade executions with prices ending in 1 or 99. Bin 2 represents trade prices ending in 2 or 98 and so on up to bin 49 which represents trades ending in 49 or 51. The 2nd column represents to sum of shares for each trade in each bin and the 3rd column represents the sum of the trade value (tradePrice * size) for each bin. With this information, it is possible to estimate the gain or loss for Investor A, Investor B and HFT.

For the first bin, Investor A gains 1/100th of a cent per share traded or the product of the number of shares and 0.0001. For bin 2, Investor A gains 2/100ths of a cent per share. For all bins, Investor B will lose the bid/ask spread (we assume the best possible case of a 1 cent spread), which is the product of the number of shares and 0.01. Using our simple model where HFT and Investor A split the penny lost by Investor B, we can attribute the gain to HFT as being the negative of Investor B's loss, minus the Investor A's gain. For July 19, 2012, trades with sub-penny prices (excluding 1/2 cent) consisted of 415 Million shares and had a dollar value of $14 billion. Out of those $14 billion worth of trade executions, Investor A group as a whole, received price improvement totalling $570,016, while  Investor B group lost $4.1 Million, and HFT made a profit of $3.6 Million.


The Anomaly

So what is the anomaly?

If we separate the price bins back out (instead of combining bin 1 and 99, 2 and 98 and so on) and then plot the total shares and $value for each bin, the resulting graph shows a remarkably consistent mirror image between the two price bins previously combined. That is, the number of  shares and $ value of trades with prices ending in #.##01 will be remarkably similar to trades with prices ending in #.##99. Share counts and $ value of trades with prices ending in #.##02 will mirror trades with prices ending in #.##98. Likewise for 3 and 97, 4 and 96 and so on all the way to 49 and 51.

Step through the charts below which show a variety of trading days from the flash crash, to pre-holiday trading session, to recent days: every one shows a consistent mirror image. The wiggles in the lines to the left of center match the wiggles in the lines to the right of center. The center (0 on the x-axis, between 95 and 5 ) is a whole cent boundary.

Each image also includes a pie chart inset showing the break down of the $ value of trades in various price bins. These charts show, for example (see red wedge), that 20% to 36% of all price improvements were just 1/100th of a cent from the next whole penny. That means Investor A got a whopping price improvement of 1/100th of a cent per share, while HFT kept the other 99/100 of a cent per share.

A lot like robbing Peter of $100, paying Paul $1 and pocketing $99.




Reg NMS has much to say about sub-penny prices (does the SEC even read existing rulings before charging ahead with new regulations?).


Page 29:

The Sub-Penny Rule (adopted Rule 612 under Regulation NMS) prohibits market participants from displaying, ranking, or accepting quotations in NMS stocks that are priced in an increment of less than $0.01, unless the price of the quotation is less than $1.00. If the price of the quotation is less than $1.00, the minimum increment is $0.0001. A strong consensus of commenters supported the sub-penny proposal as a means to promote greater price transparency and consistency, as well as to protect displayed limit orders. In particular, Rule 612 addresses the practice of "stepping ahead" of displayed limit orders by trivial amounts. It therefore should further encourage the display of limit orders and improve the depth and liquidity of trading in NMS stocks.


Page 212:

To address this concern, Rule 612 as proposed would have prohibited any national securities exchange, national securities association, ATS, vendor, or broker-dealer from displaying, ranking, or accepting from any person a bid, offer, order, or indication of interest in an NMS stock priced in an increment less than $0.01 per share. This restriction would not have applied to any NMS stock the share price of which is below $1.00.

The proposed rule was designed to limit the ability of a market participant to gain execution priority over a competing limit order by stepping ahead by an economically insignificant amount. In issuing the sub-penny proposal, the Commission cited research performed by OEA showing a high incidence of sub-penny trades that cluster around the $0.001 and $0.009 price points. The OEA study concluded that this phenomenon resulted from market participants attempting to step ahead of competing limit orders for the smallest economic increment possible. In the Proposing Release, the Commission pointed to a variety of additional problems caused by sub-penny quoting, including the following:

• If investors' limit orders lose execution priority for a nominal amount, investors may over time decline to use them, thus depriving the markets of liquidity.

• When market participants can gain execution priority for an infinitesimally small amount, important customer protection rules such as exchange priority rules and NASD's Manning rule could be rendered meaningless. Without these protections, professional traders would have more opportunity to take advantage of non-professionals, which could result in the latter either losing executions or receiving executions at inferior prices.

• Flickering quotations that can result from widespread sub-penny pricing could make it more difficult for broker-dealers to satisfy their best execution obligations and other regulatory responsibilities. The best execution obligation requires a broker-dealer to seek for its customer's transaction the most favorable terms reasonably available under the circumstances. This standard is premised on the practical ability of the broker-dealer to determine whether a displayed price is reasonably obtainable under the circumstances.

• Widespread sub-penny quoting could decrease market depth (i.e., the number of shares available at the NBBO) and lead to higher transaction costs, particularly for institutional investors (such as pension funds and mutual funds) that are more likely to place large orders. These higher transaction costs would likely be passed on to retail investors whose assets are managed by the institutions.

• Decreasing depth at the inside also could cause such institutions to rely more on execution alternatives away from the exchanges and Nasdaq that are designed to help larger investors find matches for large blocks of securities. Such a trend could increase fragmentation of the securities markets.


Page 219:

Moreover, the Commission agrees with the many commenters who believe that Rule 612 will deter the practice of stepping ahead of exposed trading interest by an economically insignificant amount. Limit orders provide liquidity to the market and perform an important price-setting function. The Commission is concerned that, if orders lose execution priority because competing orders step ahead for an economically insignificant amount, liquidity could diminish. As one commenter, the Investment Company Institute, stated, "[t]his potential for the increased stepping-ahead of limit orders would create a significant disincentive for market participants to enter any sizeable volume into the markets and would reduce further the value of displaying limit orders."


Nanex Research